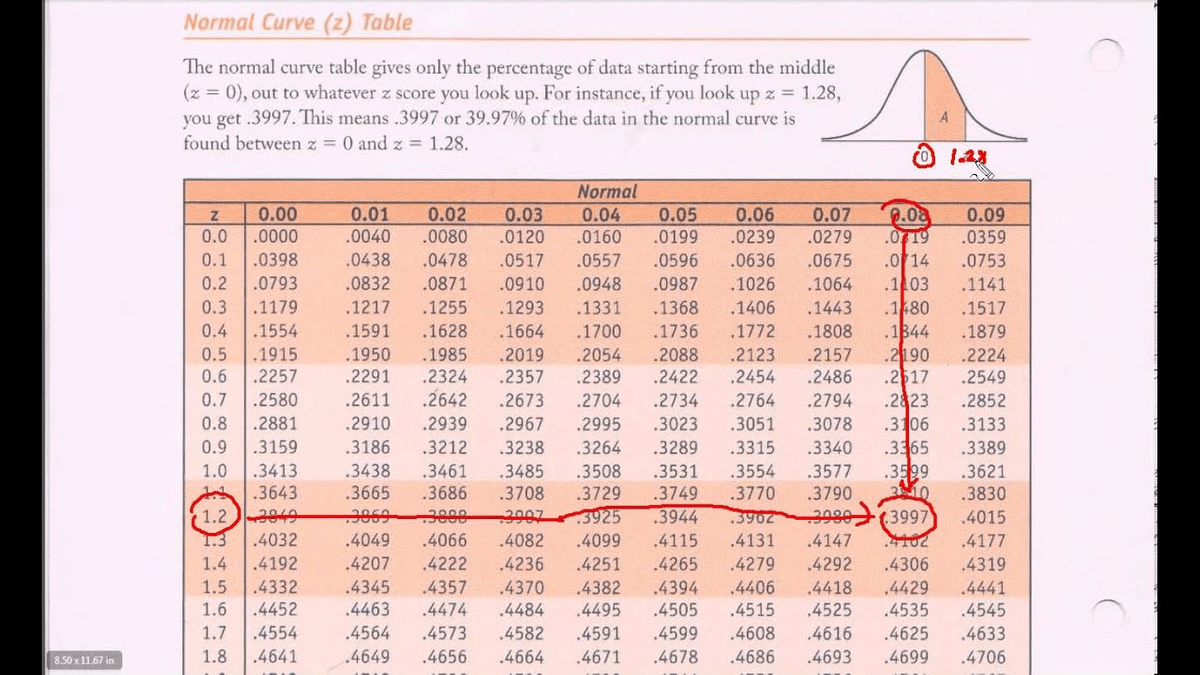
Z-scores allow for the calculation of area percentages (also called proportions or probabilities) anywhere along a standard normal distribution curve (and, consequently along the corresponding normal distribution). What do we do when the value does not fall at an Empirical Rule subdivision? By using z-scores, we have the ability to locate a percentage (or area) under a standard normal distribution at any location. These subdivisions are fine for determining percentages as long as we are dealing with values that fall at these exact subdivision locations.
We have seen that the Empirical Rule (68% - 95% - 99.7%) subdivides the area under a normal distribution into sections with widths of one standard deviation. The fact stated above is the reason we can find an area over an interval for any normal curve by finding the corresponding area under a standard normal curve (with a mean of 0 and a standard deviation of 1). (The term 'area' will refer to 'area percentage'.) For example, the area percentage to the right of 1.5 standard deviations above the mean is identical for all normal curves.
Areas under all normal curves are related.